
If you are provided with a set of discrete data, actually there is no universal agreement on selecting quartile values. This leads to a lot of confusion as different teachers use different methods to teach, and hence students sometimes get a different answer from the standard answer.
However, in the case of most common public exam in UK and US, like GCSE/IGCSE/SAT, the following method is used, so if you are taking exam from these countries, please be sure you understand and able to apply the following method.
Step 1: Arrange the set of discrete data in ascending order. (VERY IMPORTANT!)
Step 2: Use the median to divide the data set into two halves. If there are an odd number of data points in the original data set, do not include the median in either half, however, if there are an even number of data points, split the data set exactly in half.
Step 3: The median of the lower half of the data set is the lower quartile. The median of the upper half of the data set is the upper quartile.
So let's look into four examples involving different number of data points.
Example 1 (12 data points)
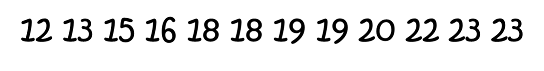
The median is in the middle between 18 and 19. (So it is 18.5) The number of data points is an even number, so let's split it into half.
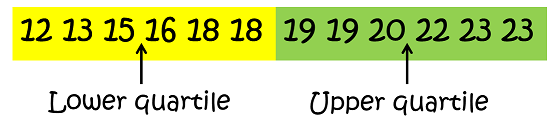
So the lower quartile is 15.5 and the upper quartile is 21.
Example 2 (13 data points)
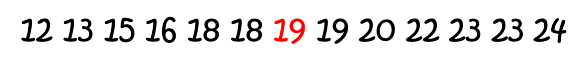
The median is the first 19 (which I colored in red), since there are an odd number of data points, let's split the data set without including the median.

So the lower quartile is 15.5 and upper quartile is 22.5.
Example 3 (14 data points)
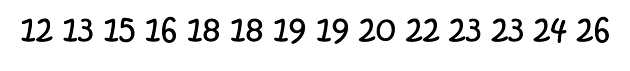
The median is between the two 19. So let's split the data set into half.
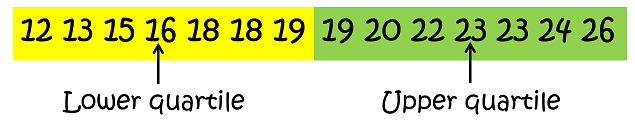
Lower quartile is 16 and Upper quartile is 23.
Example 4 (15 data points)
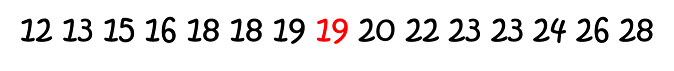
The median is the second 19 (which I colored in red), since there are an odd number of data points, let's split the data set without including the median.

So lower quartile is 16 and upper quartile is 23.
Hope this post can clear up all the confusion. And it is your time to do some exercises (answers at the bottom):
Find the lower quartile and upper quartile for the following data sets.
Set 1:
7 18 18 24 24 26 28 32 33 33 33 34 35 39 40 40 40 40 40
Set 2:
27 29 29 32 32 33 35 36 40 41 44 48 49 53 53 56 56 56 58 60 61 64 65 72 73 81
Answers:
Set 1: Lower quartile: 24 Upper quartile: 40
Set 2: Lower quartile: 35 Upper quartile: 60
Congratulations @physicsandmath! You have completed some achievement on Steemit and have been rewarded with new badge(s) :
Click on any badge to view your own Board of Honor on SteemitBoard.
For more information about SteemitBoard, click here
If you no longer want to receive notifications, reply to this comment with the word
STOPCongratulations @physicsandmath! You have completed some achievement on Steemit and have been rewarded with new badge(s) :
Click on any badge to view your own Board of Honor on SteemitBoard.
For more information about SteemitBoard, click here
If you no longer want to receive notifications, reply to this comment with the word
STOP